May 1, 2018: The Commutative Property and Multiple-Digit Multiplication

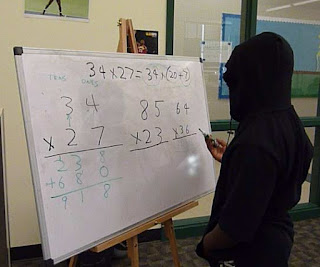
The last two weeks have been quite productive.  We now have four families who come on a fairly regular basis, and at times we have had nine kids at our weekly teaching sessions.  Most of the kids are between nine and twelve years old, but we do also have a six year old girl and a five year old boy.  The older kids are starting to tackle multiple-digit multiplication, as well as the commutative and distributive properties of arithmetic.  This is exciting because once our kids have mastered these things, we will be ready for division.  And after that, I look forward with great excitement to spending some serious time teaching general strategies for solving word problems.  Below you can see a few video clips of our last week's teaching session, where the kids demonstrated the commutative property of addition and multiplication, and one of our kids demonstrated double-digit by single-digit multiplication:

We now have a YouTube channel, for those who want to share our progress videos without having to go to our blog.  And hopefully within the next month I will upload a picture of the entire North Portland teaching group.

*  *  *

And now, for the answer to the question I asked in the last post.  The smaller bottle of parsley sells for $2.04 and contains a half ounce of parsley.  The larger  bottle contains 0.875 ounces and sells for $4.05.  On the bigger bottle is a large sticker which reads in bold letters, "3x More" and in much smaller print, "Than our 0.25 ounce size".  While the label does not contain false information, it does give a false impression when placed next to the half-ounce bottle of parsley.  Indeed, it gives two false impressions - first, that the larger bottle contains three times more parsley than the half-ounce bottle, and second, that buying one of the larger bottles is a better buy than buying two of the smaller bottles.  To verify that buying two of the smaller bottles is a better buy, just calculate the unit price as follows:

Unit price = price of bottle divided by amount of parsley in bottle

This is an example (admittedly almost trivial) of how a nation that is increasingly lacking in numeracy is becoming increasingly vulnerable to being cheated in small and large ways.  I hope to explain this topic in more detail in future posts.